Exploring Watkins' Objection
by Terence McKenna
As I understand the Watkins' Objection it comes down to saying that the method used to evaluate the simple wave does not in fact preserve and quantify the qualities that it was intended to preserve and quantify. Therefore it is important to carefully retrace and understand the process by which the quantification of the simple wave was done. My attack on the problem began with an examination of the simple wave of Figure 1. This figure is the module out of which the timewave is constructed and its quantification is the basis therefore of the predictions made concerning the ebb and flow of novelty.

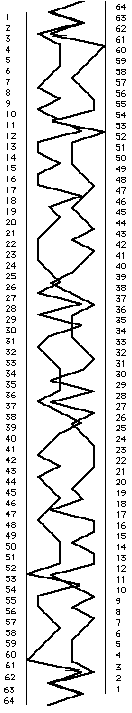
Figure 1

The first step seemed to me to be to isolate and assign numerical values to the lines which form the simple wave. Thirteen discrete line types comprise any simple version of the wave. These thirteen lengths are displayed on and off grid in Figure 2:

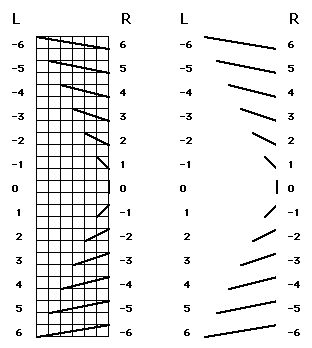
Figure 2

As these lengths are always discrete units, we can assign to them values which are ascending integers. The values of Figure 2 allow a quantification of line length. To quantify the degree and direction of skew of individual lines, one direction of skew is designated as positive, giving lines skewed in that direction positive values. Lines skewed in the opposite direction are given negative values. This gives values adequately preserving and quantifying line length and direction of skew. The values labeled L in Figure 2 are used for the left side of a simple wave while the values labeled R, which are the same values with their sign reverse, are applied to the right side of any simple wave. The sign is important only in combining values across scales but is ignored in the final graphing of combined values, either set of values may be applied to either the right or left side. However, whichever schema is chosen must then be followed throughout. Figure 3 represents the version of these values that we have used for the simple graph.

In the same fashion that similar structures on the right and left side of the wave are given equal values of opposite signs in order to preserve their symmetry, so too the symmetry between the top half and the bottom half of the wave is preserved by a similar operation: The midpoint of the wave is a switch over point where signs are reversed for the 32 values that are the last half of the wave. This move preserves symmetry as those values switch from one side of the wave to the other. This is the famous "twist" that Watkins has claimed is not a rational move. More on this further on.
Take a look at the values that enter into the valuation of skew:

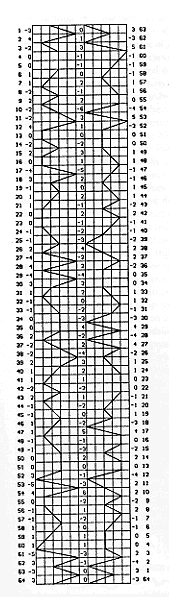
Figure 3

It is important to note that the valuations in Figure 3 are valuations of the simple wave on the smallest scale of a single complex wave. The relative proportions of the three levels in the complex wave are preserved and quantified by multiplying the valuations of the linear scale in the appropriate way. To assign a value to a position on the trigramatic scale, the valuation of that position on the linear scale (Figure 3) is multiplied by three because the trigramatic scale is three times larger than the linear scale. In a similar manner, the hexagramatic positions are assigned a valuation by multiplying their linear-level valuations by six, again because the hexagramatic scale is six times larger than the linear. Figure 3 uses the value scheme in Figure 2 and is the version of value assignments we have used in all our calculations. For a graphic representation of the relation of the three levels in a complex wave see Figure 11.

Note that in Figure 3 all parallel lines, regardless of the distances separating them, reduce to zero. Thus, while the operations discussed so far have allowed quantification of skew direction, proportional ratios of the wave parts, and the degree of departure from the parallel state, they have not provided a quantified account of the fluctuating distances between the two parameters of the wave. The procedure for obtaining these values is similar to, but distinct from, the procedures outlines above.

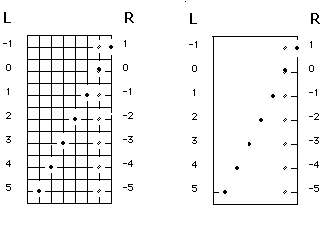
Figure 4

Figure 4 shows the seven types of divergence, congruence, and overlap which points in the simple wave may display. The two possible assignments of positive and negative numbers are shown to the right and left sides in Figure 4. We have chosen to use the right-hand schema to preserve the intuition that overlap tends to carry a situation toward the zero state rather than away from it.

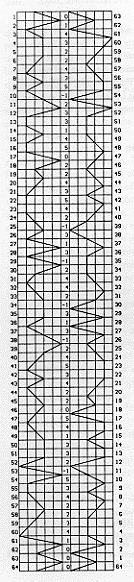
Figure 5

Figure 5 shows the values this series of point assignments generates when applied to the simple wave. When the valuations for skew, parallelism, and relative proportion have been combined in the manner detailed above the following graphic evaluation of the simple wave is the result:

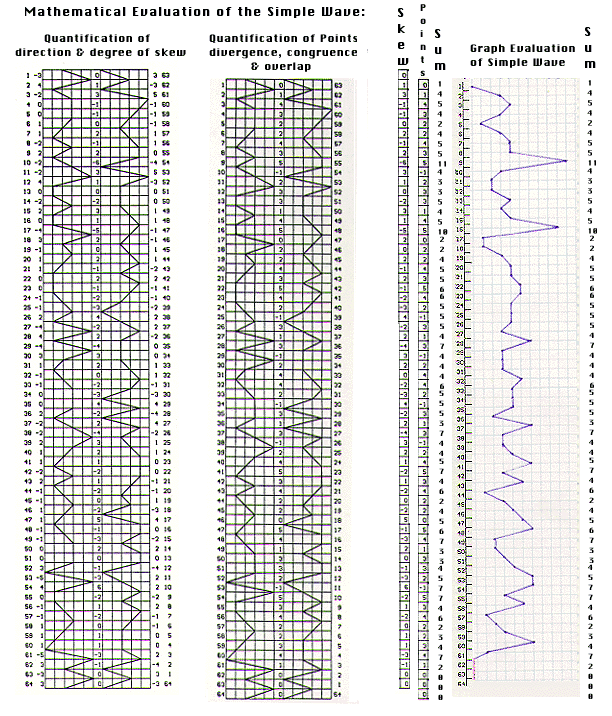
Figure 6

Figure 6 brings us to the crux of the Watkins Objection. Watkins is challenging the method by which the simple bi-directional wave of Figure 1 is converted into the figure at the extreme right in Figure 6. This is a central objection since that figure is the modular foundation of the complex wave and of the fractal hierarchy that is built upon it. Let's take a look at the simple wave and its mathematical reduction side by side. The reduction preserves all of the most important intuitions that guided the construction of Novelty Theory; in particular it preserves the idea that the area of closure in both the simple and the complex wave should quantify as zero. It is this unique zero point that gives the theory its eschatological properties and it was the centrality of this concept that lead to naming the software "Timewave Zero".

Figure 7

The skew components maintain their symmetry relative to the center of the wave by being numerically identically but of opposite sign.

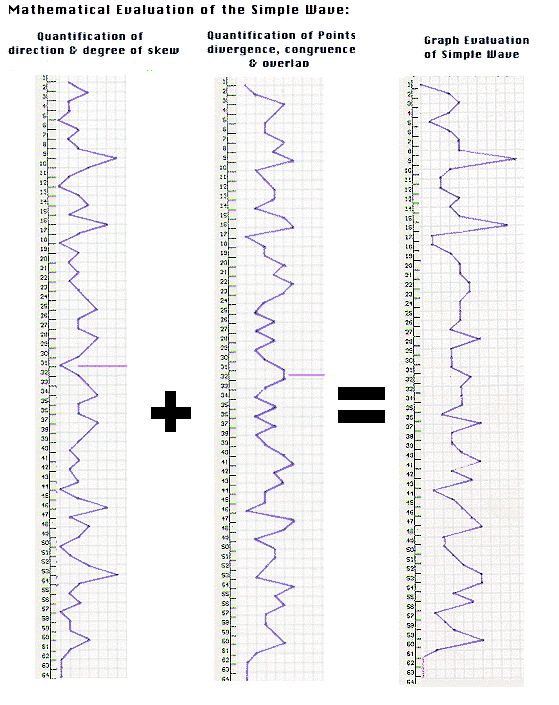
Figure 8

The point evaluations also have symmetry. The axis of symmetry of both graphs is indicated by a violet line. The one position offset of the two sets of symmetrical data points gives rise to the asymmetry of the final combined valuation. This offset occurs because Skew etc. is an evaluation of one portion of the wave to a portion directly across from it, while Point/Distance etc. is an evaluation of the transition between one portion of the wave and the portion next to it.

In order to fully understand the Watkins'' Objection it is necessary to construct a mathematical evaluation of the simple wave without the inclusion of the "twist" that Watkins is opposed to. When this is done the follow mathematical reduction takes place:

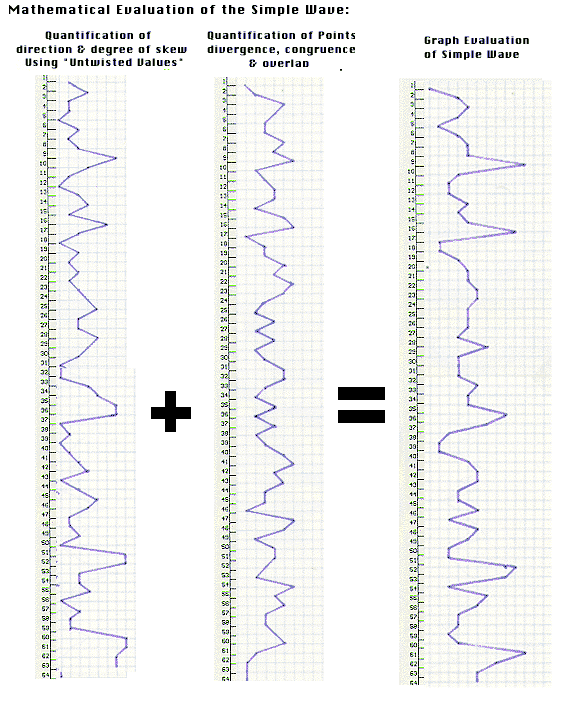
Figure 9

We are now in a position to place the simple wave, the original valuation and the valuation implied by Watkins' Objection side by side for comparison.

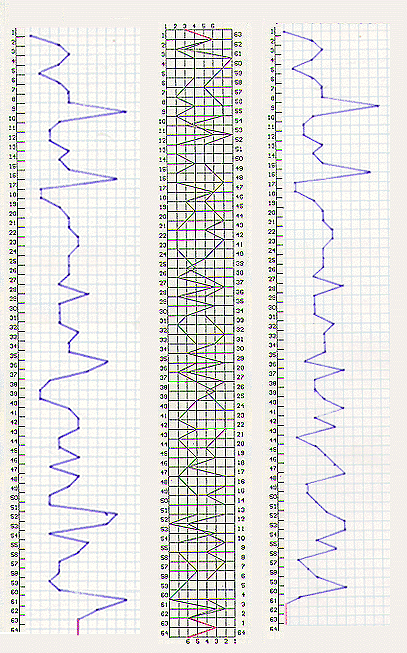
Figure 10

When this comparison is carried out I maintain that all basis for Watkins' objection to the "twist" is revealed as unfounded. With the twist removed as in the valuation to the left of the simple wave it is clear that the reduction fails to reflect a primary intuition of Novelty Theory; that closure will quantify as zero. This original and primary intuition is not preserved in the Watkins' valuation. Examine instead the valuation on the right, this is the standard valuation, generated by the inclusion of the "twist" in the method of evaluating the simple wave. this method preserves the intuition that closure will always quantify as zero. Hence the original method is to be preferred and the Watkins' Objection is answered.

QED

As for the assertion of Watkins to the effect that:
"As a result of the shifting positive and negative signs, distinct bits of the 'three level complex wave' with identical geometric/graphical 'shape' and orientation will be valued differently when the formulated 'collapse' is performed."

I can only reply that he who asserts must prove. I have sought and not been able to find such "distinct bits". Finally, if we accept that the Watkins Objection has been overcome, Figure 11 shows the way in which the standard quantified reduction of the simple wave the the far right in Figure 6 is used to built up the quantified complex wave that is the basis of Novelty theory.

Figure 11

Go Hyperborea.
McKenna home page